What is Lami’s Theorem?
In analyzing of the force problems, sometimes we require to evaluate the effect of a force in a certain direction of our interest. This is done by resolving the forces in required directions.
Resolution of a force is just an opposite process of addition of forces. The forces formed after splitting are called components of forces. Lami’s theorem plays a vital role in resolution of forces by analytical methods.
Lami’s theorem is a trigonometric expression which is extensively used for solving numerical problems of force system.
Lami’s theorem is a modified form of sin law.
Use of Lami’s theorem
Lami’s theorem is very familiar and frequently used tool to solve the numerical problems of a multiple force system.
Consider about a point O on a body which is acted upon by 3 co-planer, concurrent and nonlinear forces ( \vec {P} ), \ ( \vec {Q} ) and ( \vec {R} )
Let, magnitude of above forces are ( P ), \ ( Q ) and ( R ) which are simultaneously acting on a body at point O as shown in the space diagram.
Since body is in static equilibrium. Therefore, ( P + Q + R ) = 0
By triangle law of forces we can draw a triangle ( \triangle ABC ) with sides proportional to the magnitude of above forces.
In ( \triangle ABC ) side AB is representing the force ( \vec {P} ) , side BC is representing the force ( \vec {Q} ) and side CA is representing the force ( \vec {R} )
By geometry of vector diagram we can find the interior angles of the triangle ( \triangle ABC ) .
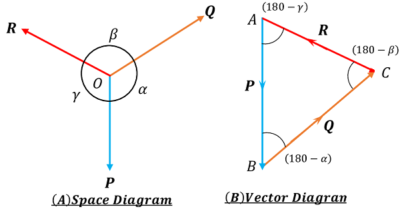
Now, if we apply sin law, we can write –
\left [ \frac {R}{\sin \left (180 - \alpha \right )} \right ] = \left [ \frac {P}{\sin \left (180 - \beta \right )} \right ] = \left [ \frac {Q}{\sin \left (180 - \gamma \right )} \right ]
This relation is known as Lami’s equation.
What is Sin Law?
Sin law establishes a trigonometric relation between magnitude of sides and opposite interior angles of a triangle. It states that, the ratio of magnitude of a side and sin of the interior angle opposite to that side of a triangle is constant.
Proof of sin law
Consider about a triangle ( \triangle ABC ) with sides ( AB = c ), \ ( BC = a ) and ( AC = b ) as shown in figure.
Perpendicular AD is drawn from the vertex A upon side BC such that it intersects BC at D or its extension as shown in figure.
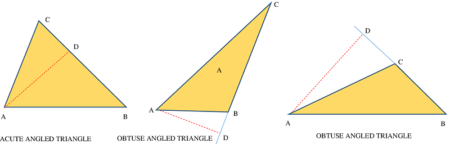
Then, AD = \text {Altitude of triangle} = h
From definition of sin functions, we have –
\sin B = \left ( \frac {h}{c} \right )
And, \quad \sin C = \left ( \frac {h}{b} \right )
Or, \quad h = c \sin B \quad and \quad h = b \sin C
Comparing above relations, we get –
h = c \sin B = b \sin C
Or, \quad \left ( \frac {c}{\sin C} \right ) = \left ( \frac {b}{\sin B} \right ) ……… (1)
BD is drawn perpendicular to AC which intersects AC or its extension at D as shown in figure.
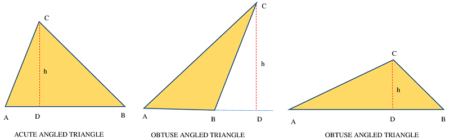
Then, \quad BD = \text {Altitude of triangle} = h
Again, from definition of “sin functions”-
\sin A = \left ( \frac {h}{c} \right )
And, \quad \sin C = \left ( \frac {h}{a} \right )
Or, \quad h = c \sin A \quad and \quad h = a \sin C
Comparing above relations, we get –
\left ( \frac {c}{\sin C} \right ) = \left ( \frac {a}{\sin A} \right ) ……… (2)
By combining relation (1) and (2), we get –
\left ( \frac { \sin A }{ a } \right ) = \left ( \frac { \sin B }{ b } \right ) = \left ( \frac { \sin C }{ c } \right )
This relation is known as sin law. It is universally applicable for all type of triangles.
Sin Law is frequently used in solving numerical problems in science and engineering.
